Convolution Theorem Laplace Transform Examples

The interval at which the DTFT is sampled is the reciprocal of the duration of the input sequence. Let C 1 C 2 be constants.

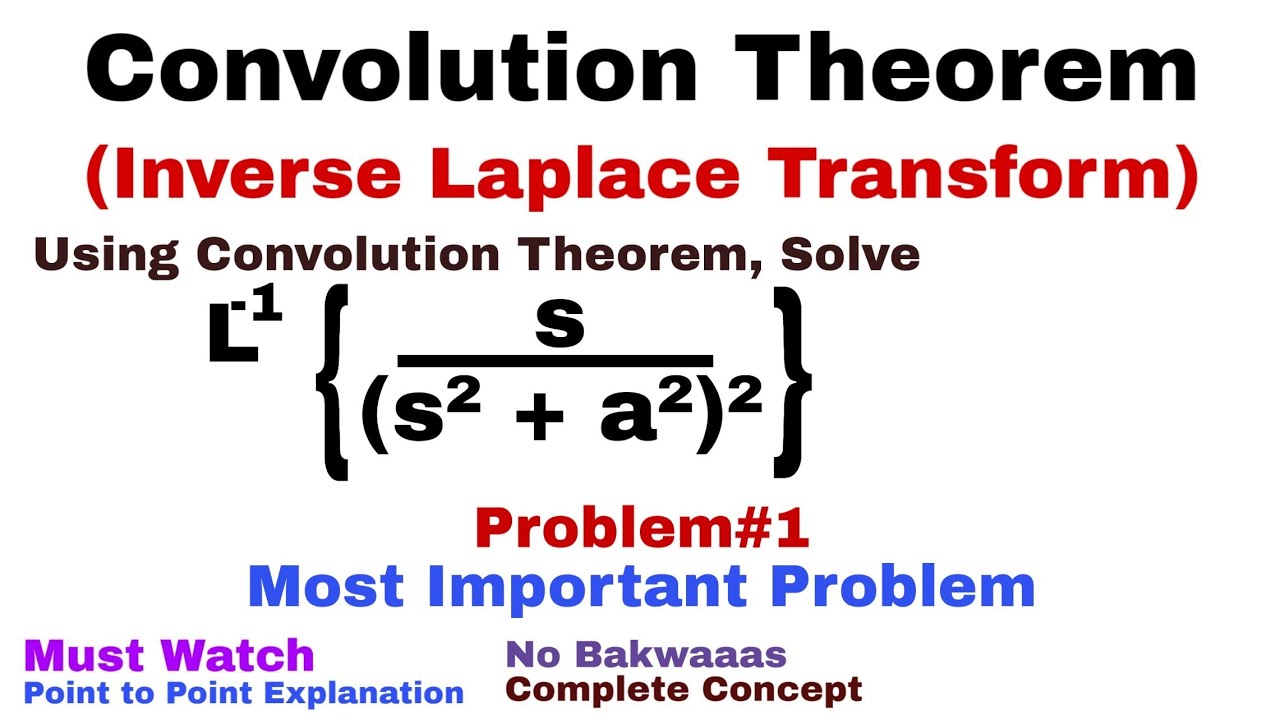
Convolution Theorem Laplace Transforms Example Problem 1 Youtube

Mathematically it has the form.

. Concept of Z-Transform and Inverse Z-Transform Z-transform of a discrete time signal xn can be represented with XZ and it is defined as. The inverse Laplace transform operates in a reverse way. Where s is the parameter of the Laplace transform and Fs is the expression of the Laplace transform of function ftwith 0 t.

In mathematics the Laplace transform named after its discoverer Pierre-Simon Laplace l ə ˈ p l ɑː s is an integral transform that converts a function of a real variable usually in the time domain to a function of a complex variable in the complex frequency domain also known as s-domain or s-planeThe transform has many applications in science and engineering because. In this section we will examine how to use Laplace transforms to solve IVPs. Z-Transforms ZT Z-Transforms Properties.

That is to invert the transformed expression of Fs in Equation 61 to its original function ft. Signals and Systems Resources. The advantage of starting out with this type of differential equation is that the work tends to be not as involved and we can always check our answers if we wish to.

Ft gt be the functions of time t then First shifting Theorem. The main properties of Laplace Transform can be summarized as follows. In mathematics the discrete Fourier transform DFT converts a finite sequence of equally-spaced samples of a function into a same-length sequence of equally-spaced samples of the discrete-time Fourier transform DTFT which is a complex-valued function of frequency.

Signals and Systems - Discussion. Signals and Systems - Resources. The examples in this section are restricted to differential equations that could be solved without using Laplace transform.

Z-transform may exist for some signals for which Discrete Time Fourier Transform DTFT does not exist. The time function ft is obtained back from the Laplace transform by a process called inverse Laplace transformation and denoted by -1.

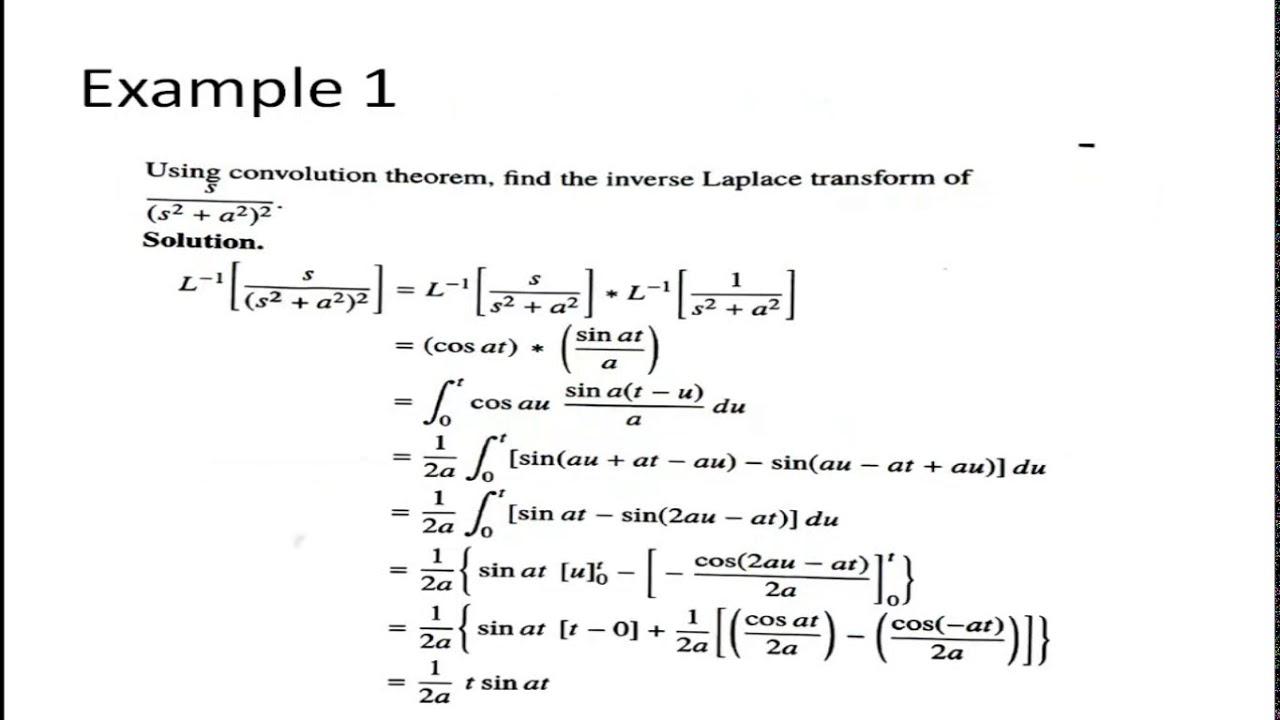
B Tech S2 S4 Laplace Transforms Convolution Theorem Youtube

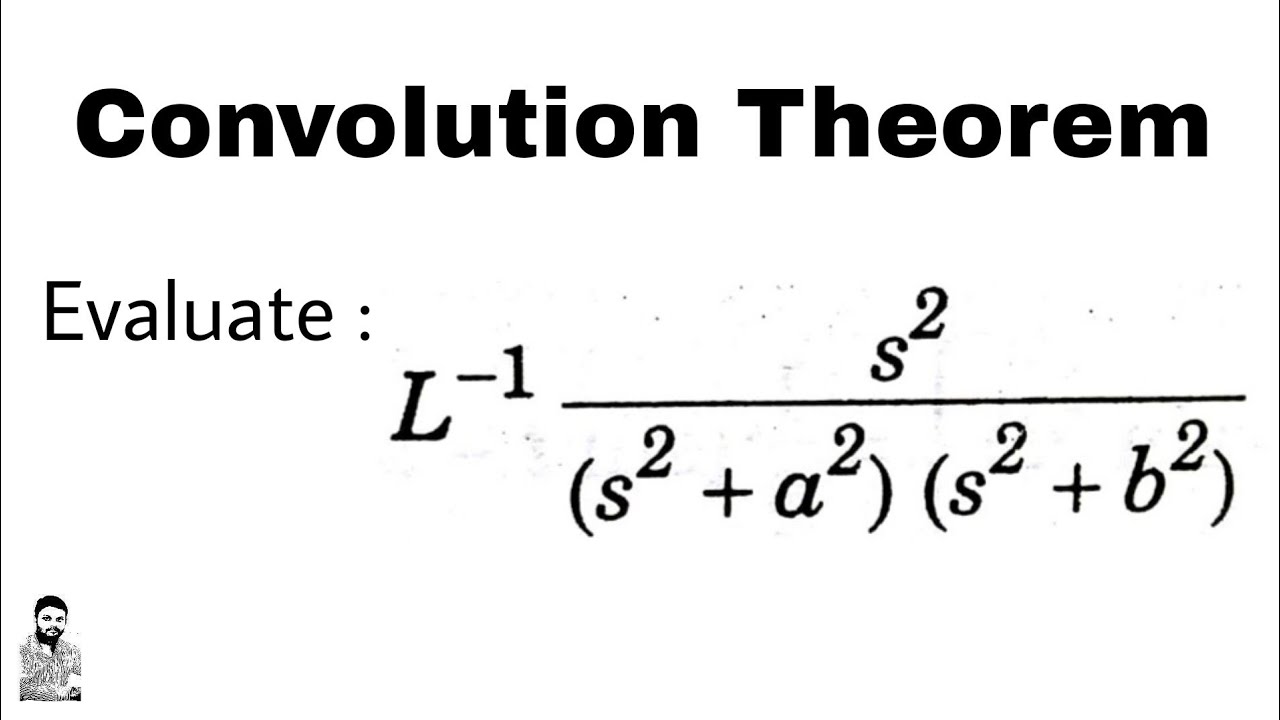
20 Convolution Theorem Problem 2 Inverse Laplace Transforms Youtube

Using The Convolution Theorem To Find The Inverse Laplace Transform Youtube

31 Convolution Theorem Complete Concept And Problem 1 Inverse Laplace Transform Youtube